
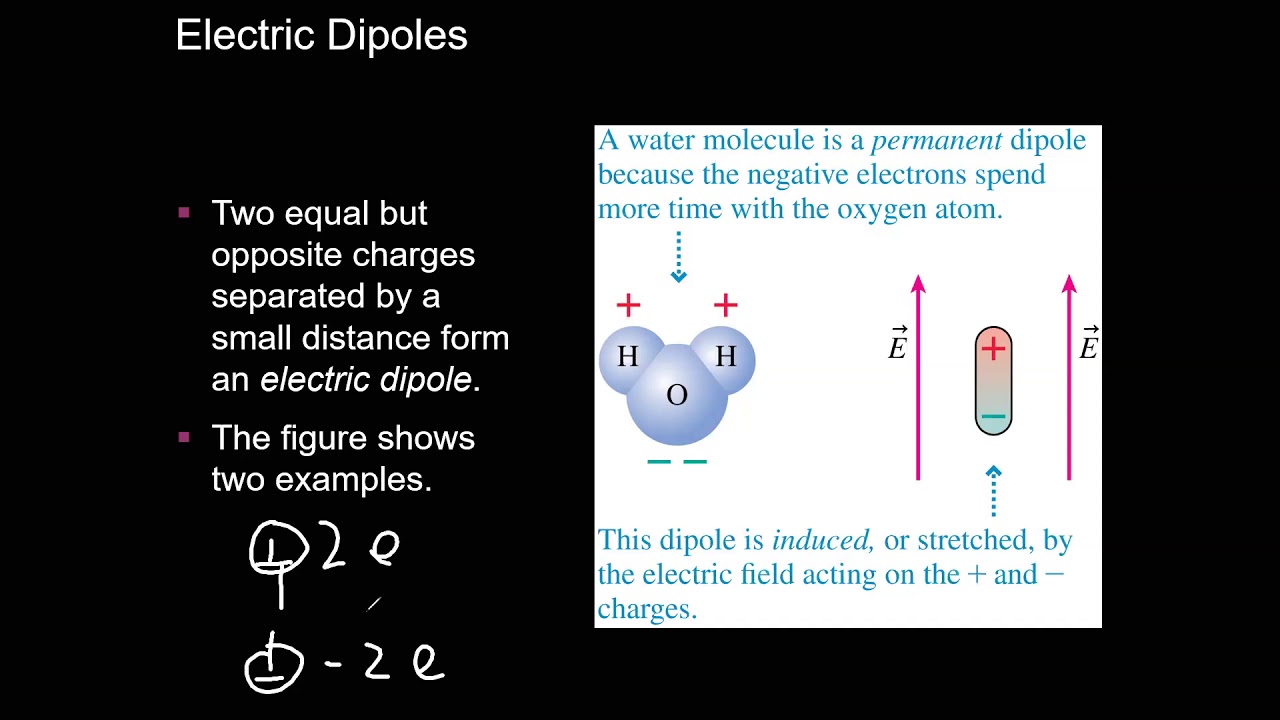

(b) When rubbed together, some negative charge is transferred to the amber, leaving the cloth with a net positive charge. Only a tiny fraction of the charges are involved, and only a few of them are shown here. (a) Both the amber and cloth are originally neutral, with equal positive and negative charges. If you comb your hair on a dry day and then put the comb close to a thin stream of water coming out of a faucet, you will find that the water stream bends toward (is attracted to) the comb ( Figure 5.2).įigure 5.5 When materials are rubbed together, charges can be separated, particularly if one material has a greater affinity for electrons than another. Another example occurs if you take a woolen sweater off quickly-you can feel (and hear) the static electricity pulling on your clothes, and perhaps even your hair. You have probably experienced the phenomenon of static electricity: When you first take clothes out of a dryer, many (not all) of them tend to stick together for some fabrics, they can be very difficult to separate. Let’s take a look at some of these activities and see what we can learn from them about electric charges and forces. But you have also most likely experienced electrical effects in other ways, maybe without realizing that an electric force was involved. And you have certainly seen electricity in a flash of lightning during a heavy thunderstorm. You are certainly familiar with electronic devices that you activate with the click of a switch, from computers to cell phones to television. Explain qualitatively the force electric charge creates.Describe the concept of electric charge.So the notion of charge quantization is really a fundamental thing- it says that all particles we discover with electric charge will have a charge which is an integer multiple of the electron's charge (for quarks this is divided by 3- the actual statement is for the U(1) charge of the fundamental gauge group, and the fundamental charges are integer multiples of 1/6- all of these crazy charges are explained by an embedding in SU(5) grand unified theory).By the end of this section, you will be able to: In all modern theories of physics, charge is quantized for fundamental reasons, and magnetic monopoles necessarily exit for the same reason. This links the first two cases to the third. The other way to extend a monopole field on the complement of a sphere to the interior is to make the sphere a black hole. So the idea of quantized charge is ultimately tantamount to the existence of monopoles.
#Discrete charge of electron full#
If you have a grand-unified theory, this monopole field can extend to the interior of the sphere, so long as the full group is compact (meaning that there is no unquantized charge in the big theory). The first two points are closely related, because if you have a discrete gauge group, you can make a monopole field on the complement of a small sphere. Small charged black holes are necessarily light in a string theory or quantum gravity completion, so you can conclude that if charge is not quantized, there are very light charged particles running around. Black holes can convert charge in protons to charge in electrons, leaving behind the difference of the two charges.This is called the Callan-Rubikov effect. In standard models of grand-unification, these monopoles catalyze proton decay.If the charges are quantized, then a certain amount of vector potential over a line is equivalent to nothing, so that a certain amount of magnetic flux is indistinguishable from no flux. The vector potential is defined by the phase shift that it makes on charged particles, and it gets multiplied by the charge to turn into a phase. Magnetic monopoles require charge quantization to be consistent.But there is no observed process which takes a proton to an anti-electron, so the charges of the two are not obviously related.īut the charge on the proton and electron are related in three different ways in modern physics. This is one precise statement of the fact that anti-particles are particles going back in time. You can understand this as a consequence of the fact that everything is made of particles with definite charge, but then it leaves the question of why the antiproton and the electron have the same charge.Īll particles have opposite charge to their antiparticle, since the particle and antiparticle are always related by reversing-time and reflecting space (this is the CPT theorem). Charge discreteness is the statement that charge comes in packets which are of size 1 electron charge.


 0 kommentar(er)
0 kommentar(er)
